Quite some time has passed since I´ve bought my own training sextant and started to think about learning how to navigate by the stars. But with the new year 2020 already in full swing I´ve made a New Year´s Pledge to spend more time sailing and do sailing-related stuff by reducing the working time. Well, at least that´s the plan. Now, spending some time with my family on the Canary Island of La Palma, I indeed find some time to go on in my celestial navigation course.
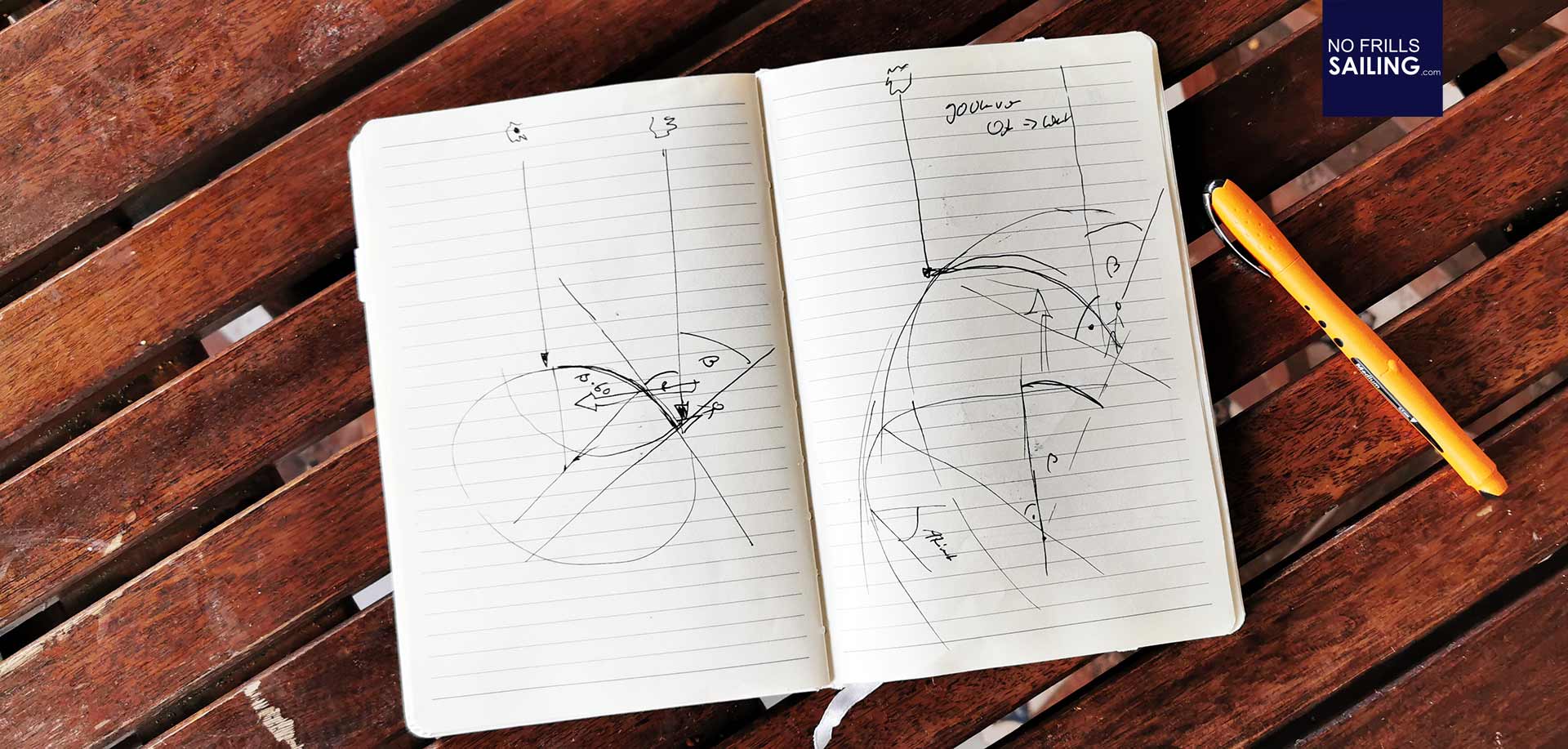
I don´t know how you prefer to learn things, but with myself it´s easy: As long as there is a story or a picture, I can internalize stuff better. Most people I know, when talking about celestial navigation, say that they don´t quite understand what exactly they are calculating and what the jumble of angles, times and numbers is all about. So, I´ve taken some Lego-toys from my kids, made a rather colorful sailor from it and put him on an admittedly very rocky “Earth” to demonstrate the first step – in essence – of what stellar navigation is all about – and what in the end is measured and calculated to determine a ship´s position.
The subsolar point
As for starters – and from feedback of other sailors – I was told that “shooting the sun” and determine one´s position by the sun is the easiest way and for beginners the recommended way of learning celestial navigation. At first, let´s understand what we are doing here: Imagine you are the Lego-man in the picture here:

The light of the Sun hits the Earth all over the surface in parallel beams. That´s crucial to understand and to visualize in order to understand celestial navigation technique. Now, we claim that we do a sun´s shoot at exactly noon: That´s 12 o´clock mid-day when the sun reaches her culmination. In this, you might imagine that there is a definitive location somewhere on the planet, where the sun´s light strikes Earth´s surface at an angle of exactly 90 degrees:

In celestial navigation this location is called the “subsolar point” or sub-point. This is crucial to understand because celestial navigation is all about this single location. Now, the rest of the story is quite easy: All you are doing celestial navigation, al the fuzz and hustle is just about one thing: To determine your distance to that sub-point of the Sun.
Determining one´s distance to the sub-point
Now the Sextant has it´s appearance. By measuring the angle between the horizon and the Sun (at exactly noon that day) you are determining the so-called “Azimut” of the stellar body (it works also with stars and of course with the Moon, the code of conduct is the same). The Azimut is just a different name for the angle between the Sun and the horizon:

Behold: Now comes the sticking point of the whole story, the one detail that made me finally understand what celestial navigation is all about. Let´s zoom out and look at Earth from a bit more afar. The angle, let´s call it “Alpha”, we just measured by means of our sextant, is the angle between Sun and horizon. The sub-point of the sun can at most add up to 90 degrees – in this case you would be exactly underneath the sun or standing on the very sub-point of the sun. So, Alpha is always less than 90 degrees. Let´s take a look on the next picture:
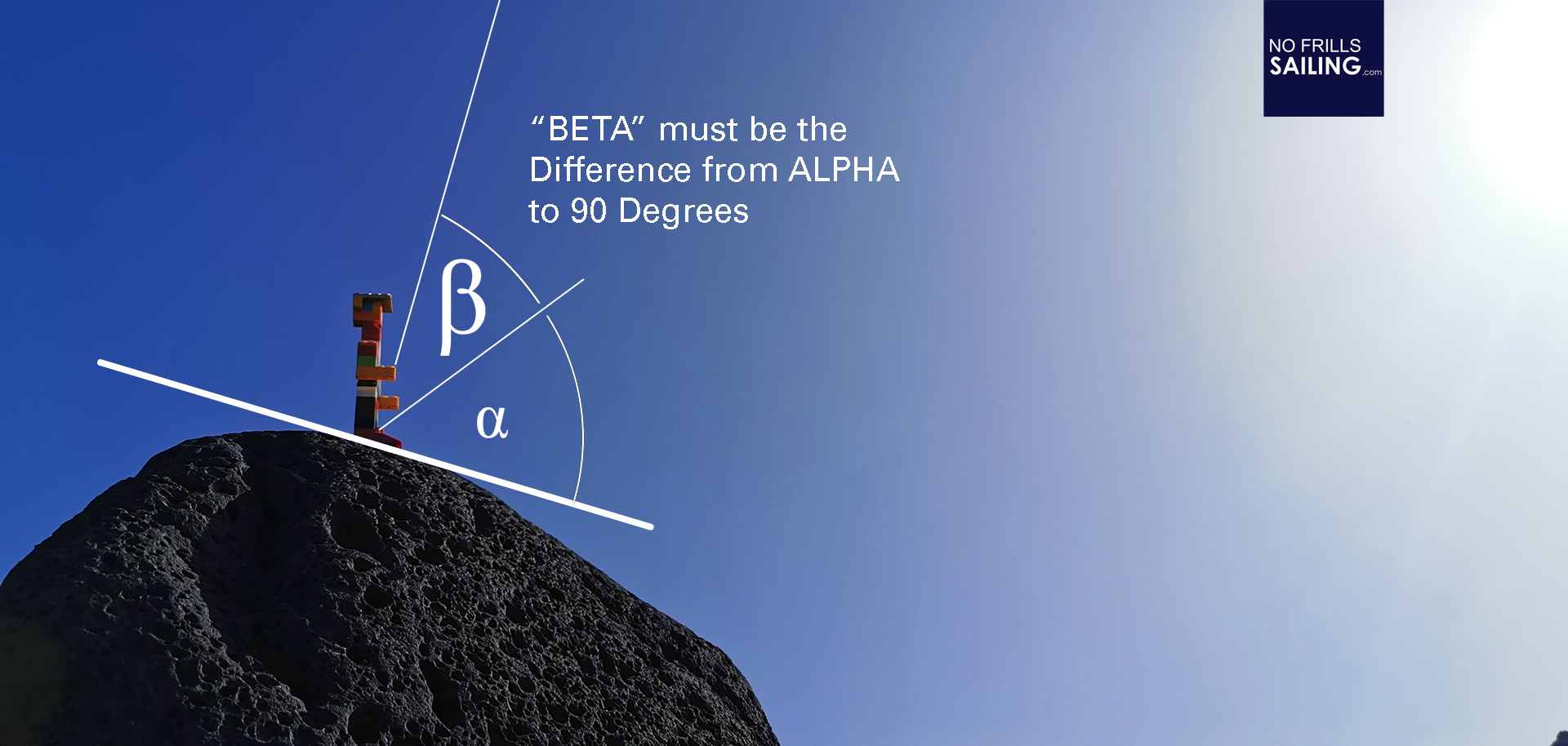
We need an angle called “Beta”. Obviously, Bet is the remainder of a simple substraction task: 90 minus Alpha. The rest is easy: Let´s transfer the standpoint of our Lego-Sailor to the imagined center of our planet Earth. We are taking all the angles with us – and now look at the very same arch which I tried to superimpose on the picture above: Can you see it?

As angles remain the same, the angle Beta resembles the very same arch which is the distance of the sub-point of our Sun to the Lego-sailor. And here´s just a simple understanding of the coordinate system of the Earth required: Because, one degree on the Earth´s 360 degrees great circle consists of 60 miles in real life. Meaning, one degree equals sixty miles. Beta – in degrees – must now be multiplied by 60 to calculate the distance in miles. So, Beta times 60 equals the distance of you to the sub-point of the sun (or any other celestial body you “shot”).
Ship´s position with two baselines
In real life, what you get is a distance which is a location somewhere on a circle around the sub-point of the Sun or any other star. This is not a position, it´s just a great circle around a point somewhere very far away – that´s millions of possible positions. What you need now is a second baseline, of course. The point in which both baselines intersect is your position. In this, there are multiple techniques. If you begin and shoot the sun, you will be waiting some hours and shoot the sun again. Now, the sub-point of the Sun “travels” at a speed of some 900 knots. You can imagine what shooting the Sun one minute late means. Or what one degree difference in measuring the angle can mean. But that is for later. After having determined another Beta, you need to “calculate back” the distance sailed and the distance the sub-point has travelled:

Where both baselines intersect, that´s the position our Lego-sailor had at a given time. Of course, skilled Navigators will shoot two stars at night at the very same time for not having to wait for the Sun´s sub-point to travel and to obtain two baselines at once. But in any case: Time is the most important thing here. It´s very, very crucial: It was the inability to measure time exactly which was prohibiting sailors from determining Longitude for Centuries – but that is another story.
For me, understanding the terminus “90 minus Alpha equals Beta” and that “Beta time 60” equals the distance to the sub-point was a major key to understanding celestial navigation. Now, let that sink in for some time before we tackle the next steps.
Related articles:
Understanding the Longitude-problem
Buying a Sextant
At Wempe in Hamburg
